Timepiece Accuracy. Part One: Sundials

This piece reflects on how perceptions of accuracy in timekeeping devices and perceptions of timepieces themselves have changed throughout history.

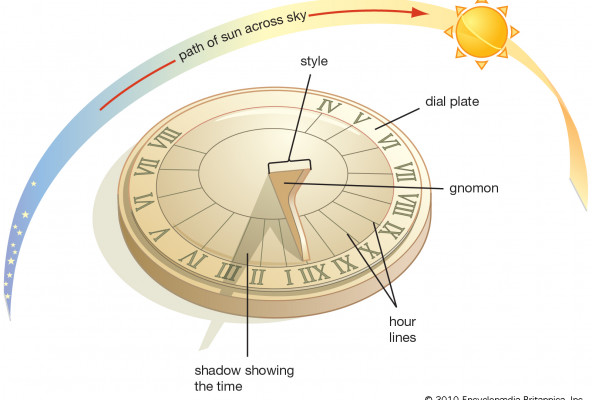
The question of accuracy in sundials might seem like a strange one at first glance, but ancient civilizations learned to tell the time according to the apparent position of the Sun. The devil is always in the details, and there are ample nuances among the sundial’s many different manifestations. First of all, there’s no one way to measure time directly according to the Sun's position.

The sundials used by the Ancient Babylonians, who can probably be credited with inventing the device, divided every day into twelve equal segments, as did the Ancient Egyptians, Greeks and Romans. This meant that each hour was shorter in winter and longer in summer. The duration of an hour would also vary depending on latitude, and the further north you were the greater the variation would be.

That's why the question of accuracy was was a rather serious one when using these sundials for timekeeping. A system using hours of equal time length throughout the year was introduced in the 13th-15th centuries, which came hand in hand with progress in mechanical clockmaking, where the use of an uneven system for timekeeping in clocks posed technical challenges for their design.

Advances in mechanical clocks influenced the sundial, which also adopted hours of equal time length, although dials were still sometimes marked according to both systems. The level of accuracy provided by sundials at the time was roughly the same as contemporary mechanical clocks, but sundials had the advantage of being able to be set to the exact time according to the position of the Sun, and they never broke.

When mean solar time with hours of equal time length was adopted, it soon became clear that the non-uniformity in the Sun's apparent daily motion across the sky needed to be factored in. It’s the reason why apparent time can be ahead of annual mean solar time by 16 minutes or behind mean solar time by 14 minutes.

This discrepancy between the two kinds of solar time is described by the equation of time, caused by the Earth's orbital eccentricity as it revolves around the Sun and the the Earth's tilted axis not being perpendicular to the plane of its orbit around the Sun.

Astronomers in Ancient Babylon are believed to have been familiar with the equation of time, but this knowledge wasn't applied in designing sundials for a long time. Astronomers and clockmakers preferred to correct clock time by tabulating the difference between apparent solar time and mean solar time as they transitioned from using apparent solar time to mean solar time.

The first sundials to use the equation of time were apparently the ones known as noon-mark sundials or meridian sundials, which didn't give the hours and were only intended to note the exact moment of 12:00 noon according to the local apparent solar time and mean solar time.

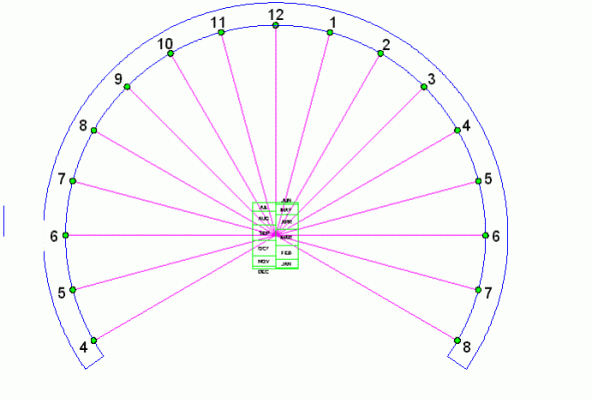
The midday line was depicted on their dials in the form of an analemma, which made it possible to supplement the midday display with a more or less accurate indication of the current day of the year. The analemmatic sundial could give the time of day throughout the entire solar day and on any day of the year, which takes the equation of time into account and has an analemma-shaped gnomon. 

The most precise modern sundials in their classic form are accurate to the nearest minute, as it takes the Sun two minutes to travel a distance equal to its own angular diameter on the Sun's apparent path known as the ecliptic, which is about half a degree, and the angular width of the shadow from the sunlight is the same size.

That's why sundial played an important role in ensuring accurate timekeeping in practice before the pendulum was invented, i.e. right up to the 18th century, the point when mechanical watches overtook sundials in terms of timekeeping accuracy.

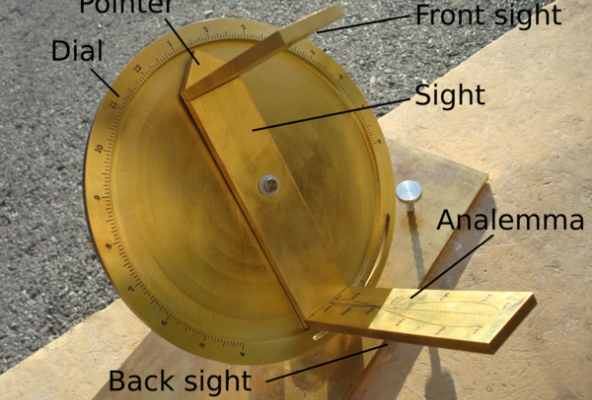
Yet many precision sundials or heliochronometers were still manufactured in the 19th century and in the early 20th century, which were  used to convert solar time to mean time and significantly improved timekeeping accuracy. It was the heliochronometer, a sundial designed to accurately determine the standard time, which allowed the accuracy of sundials to be increased.

They're designed based on the armillary sphere or equatorial sundial and Foster-Lambert's analemmatic sundial, which has evenly spaced hour lines and uses use a movable gnomon for changing the position daily to accurately indicate time of day.

The best examples of these sundials are accurate to the nearest 10 seconds. Heliochronometers began to be produced on a mass scale in the 1880s around the same time when the standard time system with time zones was adopted. Heliochronometers were no longer needed for practical purposes when the exact time began to be broadcast over the radio in the early 20th century.

In order to tell the correct standard time in a time zone (also referred to as civil time), the equation of time needs to be included in the design, i.e. an analemma needs to incorporated into the heliochronometer in some way or another.

Apart from that, the sundial needs to be corrected to account for the shift that takes place due to the leap year cycle, as the tropical year can be six hours longer than a common year, and additional adjustment may also be required on February 29 in a leap year. This last factor means that a heliochronometer's accuracy varies from year to year.

The heliochronometer is considered to have come into being on May 13, 1862, when the French engineer Paul Fléchet patented his design for an improved precision sundial (heliochronometer), based on the design of the equatorial sundial. Paul Fléchet's solar chronometer was accurate to the nearest 15 or 20 seconds.

Precise mechanical watches known as chronometers weren't in short supply at the time, but the heliochronometers built according to Paul Fléchet's design were adopted by the French railway network, apparently because sundials didn't need to be wound and wouldn't break.

In 1907, the practical design of a portable heliochronometer able to accurately determine GMT to within a minute was patented by the English astronomer and engineer George James Gibbs. He went into partnership with the businessman William Renard Pilkington to launch their production, which is where the Pilkington and Gibbs Heliochronometer sundials get their name from. 

Various different heliochronometer devices were later invented and made by astronomers and gnomonics enthusiasts, most of whom were Americans, such as James Hartness in 1916 and Symphorian Terraz in 1927, along with Frenchman Julian Paul Marie Guadet in 1938.

The precision sundials located in many cities around the world now serve more of a decorative function like art installations, and these fascinating sights always attract plenty of attention from tourists.